Deep rooted developments - carbide insert price
We demonstrated experimentally that our integrated 3C-SiCoI photonic platforms enable nonlinear and quantum light sources using waveguide-coupled elliptical microring cavities. We realized quantum interference systems, namely, time-bin entanglement using our photon-pair sources. By exploiting a wafer-scale bonding process, we envision the feasibility to fabricate on a chip large-scale-integrated cavities and on-chip interferometers. Our work thus paves the way toward future large-scale-integrated on-chip nonlinear and quantum photonic circuits based on this CMOS-compatible second-order nonlinear photonic platform. Besides, the recently demonstrated Pockels-effect-based 3C-SiC optical modulator21 can be integrated on the same quantum photonic chip to provide even more complex quantum state manipulation functionalities. Our measured SHG conversion and pair-generation generation efficiencies are, however, still below those state-of-the-art values from other on-chip second-order nonlinear photonic platforms, including LNoI38, GaAs36 and 4H-SiC19. The work reported in those platforms are based on resonators supporting loaded Q factors of \({10}^{5} \sim {10}^{6}\). We attribute our limited \({Q \sim 10}^{4}\) to our un-optimized fabrication process, where the waveguide sidewall roughness resulting from the dry etching causes significant scattering losses. Given our near-vertical waveguide sidewall slope of \(86^{\circ}\), we can fabricate thicker 3C-SiC devices with a wider waveguide width, which can increase the waveguide confinement and reduce the sidewall scattering loss. The scattering losses caused by the crystal lattice defects within the 3C-SiC film can limit the cavity Q factor as well. Those defects are generated during the epitaxial process because of the large (20%) lattice mismatch between the 3C-SiC and the Si substrates. We can mitigate this issue by adopting thicker epitaxial layers, which relax the crystal lattice defects due to the lattice mismatch gradually with the film thickness46,47,48, and by thinning down the films to the target thickness while removing the high-density crystal defects near the interface with the Si substrate.”
To demonstrate the potential of building a quantum system based on our 3C-SiCoI platform, we examine time-bin entanglement using our photon-pair sources. Entangled photon-pairs constitute many quantum-enabled technologies, including quantum computing and quantum cryptography42. Among various physical dimensions for entanglement, time-bin entanglement is more robust to perturbations when the photons propagate in fiber networks42,43,44,45.
We must account for the \(\bar{4}3m\) crystal point-group symmetry of 3C-SiC32 for designing a microring cavity. When the three mixing fields propagate in an arbitrary direction within the (001) 3C-SiC film, the projection of the field of the TE-mode onto the x- and y-crystal axes results in an angle-dependent effective susceptibility \({\chi }_{{eff}}^{(2)}=\cos \varphi \,\sin \varphi \,{\chi }_{{zxy}}^{(2)}\)33. The \({\chi }_{{eff}}^{(2)}\) is optimized with the waves propagating in directions \(45^\circ /135^\circ\) between the x- and y-crystal axes. The nonlinear polarization of a \(\bar{4}3m\) crystal has a \(\pi\)-phase flip with the wave propagation directions rotated by \(90^\circ\). While we may leverage this characteristic property to attain \(\bar{4}\)-QPM, as theoretically studied and experimentally demonstrated in compound III-V material systems featuring the \(\bar{4}3m\) crystal symmetry34,35,36, this method typically imposes a few-micron cavity size for microdisk resonators which may limit the Q factor34,35. The design principle also involves careful alignment of resonances with a certain mode-order difference34,35,36. These impose an overall tight tolerance for device fabrication. Figure 1c schematically illustrates our mitigation of adopting an elliptical cavity. In our scheme, to attain efficiently phase-matched nonlinear processes within microring cavities, we designed an elliptical microring cavity, with a major-to-minor-axes aspect ratio of 2 to increase the MPM interaction length along the flat arc waveguide in one \(90^{\circ}\) rotation of the fields while decreasing the reverse conversion around the two high-curvature poles. We adopted a semi-major axis \(a=30\mu m\) and a semi-minor axis \(b=15\mu m\), which corresponds to a circumference \(L=145\mu m\), to enable a smaller mode volume compared with mm-sized cavities.
Wu, I. J. & Guo, G. Y. Second-harmonic generation and linear electro-optical coefficients of SiC polytypes and nanotubes. Phys. Rev. B Condens. Matter Mater. Phys. 78, 1–10 (2008).
Fiorentino, M. et al. Spontaneous parametric down-conversion in periodically poled KTP waveguides and bulk crystals. Opt. Express 15, 7479–7488 (2007).
We fix the pump power of the TE-mode at 1575.4 nm within the through waveguide at ~0.5 mW on-chip power (see Methods) and measure the TM-polarized SHG at the drop waveguide port. Figure 3g shows the corresponding measured SHG spectrum using an optical spectrum analyzer (OSA). The full-width at half-maximum (FWHM) of the pump and of the SHG are limited to the equipment resolution of 0.02 nm. The inset shows the top-view of the SHG scattering signal captured by a CCD camera. As the SHG is a second-order nonlinear harmonic generation driven by simultaneously absorbing two fundamental pump photons, the SHG is signified by a parabolic dependence on the pump power. Figure 3h shows the measured pump-to-SHG power dependence in a log-log scale, revealing a fitted slope of \(1.98\pm 0.01\) that is consistent with the nature of the SHG. We estimate the on-chip SHG conversion efficiency to be \({\eta }_{{SHG}}=\frac{{P}_{{SHG}}}{{P}_{p}^{2}}=17.4\pm 0.2 \% {W}^{-1}\) with the maximum waveguide end-facet out-coupling loss of 5.8 dB (See Supplementary Note 7 for details). We calculate the theoretical SHG conversion efficiency as follows37:
where \({\omega }_{1(2)}\), \({\kappa }_{1(2),t}\), \({\kappa }_{1(2),e}\) and \({\varDelta \omega }_{1(2)}\) are the angular frequencies, the cavity total loss rates, the cavity external coupling rates and the angular frequency detuning from the cavity resonances of the 1575 nm pump (SHG) waves. The nonlinear coupling coefficient \(\gamma\) is given by:
Fan, T., Moradinejad, H., Wu, X., Eftekhar, A. A. & Adibi, A. High-Q integrated photonic microresonators on 3C-SiC-on-insulator (SiCOI) platform. Opt. Express 26, 25814 (2018).
Radulaski, M. et al. Scalable Quantum Photonics with Single Color Centers in Silicon Carbide. Nano Lett. 17, 1782–1786 (2017).
a Electric-field amplitude distributions of an TE00 mode at 1560 nm and of an TM20 mode at 780 nm. b Effective refractive indices of the pump mode (solid line) and of the signal/idler mode (dashed line) with different 3C-SiC film thicknesses as a function of the waveguide width. c Dispersion of the TM20 pump mode (solid line) and of the signal/idler TE00 mode (dashed line). d Calculated map of the non-degenerate difference-frequency generation (DFG) phase-matching condition.
Luo, R. et al. Optical Parametric Generation in a Lithium Niobate Microring with Modal Phase Matching. Phys. Rev. Appl. 11, 1 (2019).
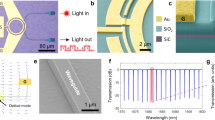
Scanning electron microscopy (SEM) images of (a) the device, (b) the zoom-in view of the sidewall, and of (c) the cross-sectional view of the waveguide end-facet. d Near-field image of the TM20 mode. e Corresponding lens-to-lens normalized transmission spectra of the device for 780 nm wavelengths (red) and 1550 nm wavelengths (black). f Zoom-in view of the aligned resonances with a Lorentzian resonance lineshape fitting of the NIR resonance. g Measured pump (black) and second-harmonic generation (SHG) (red) spectra. Inset: Top-view scattering of the SHG light. h Measured and fitted pump-to-SHG power dependence in a log-log scale.
Jerphagnon, J. & Kurtz, S. K. Maker fringes: A detailed comparison of theory and experiment for isotropic and uniaxial crystals. J. Appl. Phys. 41, 1667–1681 (1970).
For the SPDC, which is a spontaneous second-order nonlinear generation of photon pairs by down-converting one pump photon, the SPDC is signified by a linear dependence on the pump power. Figure 5d, e shows the measured power-dependence of the single-channel counts and of the photon-pair coincidence counts. We estimate the PGR inside the cavity by \({PGR}=\frac{{N}_{s}{N}_{i}}{{N}_{{si}}}\), where \({N}_{s(i)}\) are the single-channel counts from the signal (idler) flux. Figure 5f shows the estimated PGR. We observe a good linearity of the estimated PGR and obtain by linear fitting a pair generation efficiency of \(0.94\pm 0.02\) MHz mW−1 (1569–1581 nm) and \(1.32\pm 0.07\) MHz mW−1 (1562–1588 nm). We calculate the theoretical PGR assuming the triple-resonance condition as follows38:
Wu, K., Zhang, Q. & Poon, A. W. Integrated Si3N4 microresonator-based quantum light sources with high brightness using a subtractive wafer-scale platform. Opt. Express 29, 24750 (2021).
Machining grooves and shallow channels. Example: grooving ball-bearing raceways. Typically performed by tools that are capable of light cuts at high feed rates. Imparts high-quality finish.
We input both the on-resonance near-visible pump and NIR signal waves from the drop- and input-bus waveguides, respectively (see Supplementary Note 4). In each experiment, we fix the pump wavelength \({\lambda }_{p}\) at a cavity resonance around 780 nm and then tune the signal wavelength around different cavity resonances at the blue side of \(2{\lambda }_{p}\). We fine-tune the pump and signal wavelengths around their respective cavity resonances to maximize the output DFG idler power generated at the red side of \(2{\lambda }_{p}\) (see Supplementary Note 9). We fix the estimated on-chip power of pump and signal waves at 4 mW.
Furthermore, the APAC region is also expected to remain the most attractive region in terms of market attractiveness by market share index, on account of the largest volumes of cutting tool inserts consumptions expected by the region, over the forecast period, mainly driven by China. A high growth rate due to considerable industrial activity in the region is expected to contribute to the rising demand. Europe is expected to hold a significant market share in terms of both value and volume after APAC region, owing to growing automotive and other end use industries and infrastructural developments in the region.
a Schematics of a parametric down-conversion photon-pair source on a 3C-SiCoI platform. b Schematics of the Type-I phase-matching process. c Illustration of an elliptical microring cavity with the normalized effective nonlinearity \({\chi }_{{eff}}^{(2)}/{\chi }_{{zxy}}^{(2)}=\cos \varphi \,\sin \varphi\) as a function of rotation angle \(\varphi\) of the fields relative to the crystal x-axis. Purple represents the pump frequency, blue and red represent the generated signal and idler frequencies, respectively.
Enlarging a hole that already has been drilled or cored. Generally, it is an operation of truing the previously drilled hole with a single-point, lathe-type tool. Boring is essentially internal turning, in that usually a single-point cutting tool forms the internal shape. Some tools are available with two cutting edges to balance cutting forces.

Li, J. & Poon, A. W. Time-bin Entanglement Based on Integrated 3C-SiC-on-insulator Photon-pair Sources. In Proceedings of CLEO (San Jose, USA, 2023).
Dong, N., Chen, F. & Vázquez de Aldana, J. R. Efficient second harmonic generation by birefringent phase matching in femtosecond-laser-inscribed KTP cladding waveguides. Phys. Status Solidi Rapid Res. Lett. 6, 306–308 (2012).
Figure 6a illustrates the principle of generating time-bin entanglement using a free-space Franson unbalanced interferometer. The photon-pairs can simultaneously travel through the short arm together and the long arm together, which are indistinguishable. The photon-pairs can also travel through the short arm and the long arm separately, which are distinguishable. When the photons re-enter the fiber system and routed to SNSPDs through the DWDM filters, the recorded histogram of their relative arrival times is shown in the Inset. We attribute the two side peaks as the distinguishable events that the two photons propagate in different arms. The central time-bin measures the photon-pair in an entangled state as \(|\psi > =\frac{1}{\sqrt{2}}({{|SS} > }_{{si}}+{e}^{i\left({\varphi }_{s}+{\varphi }_{i}\right)}{{|LL} > }_{{si}})\), where S(L) and \({\varphi }_{s}\)(\({\varphi }_{i}\)) denote the short (long) arm and the optical phase difference between the two arms of the signal (idler) photon. To separate the central peak time-bin from the side peak time-bins, we unbalance the interferometer arms by exceeding the coherence length of the photons limited by the cavity lifetime, calculated by \({L}_{c}=\tau c\), which is 7.8 mm for our case.
Finally, there are rooms for our elliptical microring to further enhance the cavity-roundtrip nonlinear interaction coefficient \(\gamma\). For example, the ellipse of a larger \(a/b\) aspect ratio can further align the cavity along the orientation that enables the maximum \({\chi }_{{eff}}^{(2)}\) (see Supplementary Note 13). However, the design tradeoff is reducing the bending radius of the high-curvature poles by the relation \({R}_{{pole}}=\frac{{b}^{2}}{a}\). To provide further elongation while avoiding a larger bending curvature, other non-circular microring configurations can also be explored, including racetracks with Euler bending49 and Bezier bending50 designs. However, special optimization of these bending structures may be required to reduce the straight-to-curve transition loss of the two different modes at the pump/signal wavelengths simultaneously. Nonlinear and quantum light sources based on the 4-bar QPM are possible due to the point-group symmetry of 3C-SiC.
We describe the experimental setup in Supplementary Note 4. By adopting the firing of the idler channel as the gating, we measure the signal channel as a heralded single-photon source. We utilize a fiber-based 50:50 beam splitter in the signal path to test the single-photon nature. The heralded second-order coherence is characterized by40:
Kaneda, F., Suzuki, H., Shimizu, R. & Edamatsu, K. Direct generation of frequency-bin entangled photons via two-period quasi-phase-matched parametric down conversion. Opt. Express 27, 1416 (2019).
Sheng, Y. B. & Zhou, L. Deterministic polarization entanglement purification using time-bin entanglement. Laser Phys. Lett. 11, 085203 (2014).
All the data employed for this work will be made available from the authors upon reasonable request.
Lin, J. et al. Phase-Matched Second-Harmonic Generation in an On-Chip LiNbO3 Microresonator. Phys. Rev. Appl. 6, 1–6 (2016).
Niedermeier, S., Schillinger, H., Sauerbrey, R., Adolph, B. & Bechstedt, F. Second-harmonic generation in silicon carbide polytypes. Appl. Phys. Lett. 75, 618–620 (1999).
By submitting a comment you agree to abide by our Terms and Community Guidelines. If you find something abusive or that does not comply with our terms or guidelines please flag it as inappropriate.
Figure 4a–f show the measured spectra using the OSA. The dashed line in each figure denotes the degenerate \(2{\lambda }_{p}\) positions. The pump cavity resonances supporting triple-resonance DFG span from 786.17 nm to 795.69 nm, giving a pump spectral window of 9.5 nm. We observe cavity-enhanced DFG from at least five different signal cavity resonances. Pumping at a cavity resonance of 795.7 nm, we observe the maximum non-degeneracy of 97 nm. Figure 4g shows in a scatter plot the measured triple-resonance-enhanced DFG modes. However, in comparison with the numerically simulated waveguide dispersion (Fig. 2d), the observed overall spectral range for attaining triple-resonance DFG is narrower. We attribute this to fabrication-induced non-uniformities. While the measured triple-resonance DFG spectral windows are wider than those reported of similar microring cavity-based work on the LNoI37 and AlN38 platforms. We also characterize another waveguide-coupled elliptical microring with a designed waveguide width of 1000 nm (see Supplementary Note 10) that shows a blue shift of the overall triple-resonance DFG spectral range, which is consistent with the numerical simulation of the waveguide dispersion (see Supplementary Note 2). Figure 4h shows the measured power-dependence of the DFG. We pump at a 787.7 nm cavity resonance and input a signal at a 1569.2 nm cavity resonance and measure the generated idler power at the corresponding 1581.8 nm cavity resonance. In the log-log scale, the idler power has fitted slopes of \(0.96\pm 0.01\) and \(0.90\pm 0.01\) dependence on the pump and on the signal, respectively, as expected from a theoretical linear dependence.
Kuo, P. S., Bravo-Abad, J. & Solomon, G. S. Second-harmonic generation using 4-quasi-phasematching in a GaAs whispering-gallery-mode microcavity. Nat. Commun. 5, 1–7 (2014).
Photonic Device Laboratory, Department of Electronic and Computer Engineering, The Hong Kong University of Science and Technology, Clear Water Bay, Hong Kong, China
Figure 1a schematically illustrates our elliptical microring resonator as a parametric down-conversion photon-pair source on an integrated 3C-SiCoI platform. We discuss our device design principles starting from classical nonlinearity based on DFG. The zinc-blende unit cell of 3C-SiC features tensor elements \({\chi }_{{zxy}}^{(2)}={\chi }_{{xyz}}^{(2)}={\chi }_{{yzx}}^{(2)} \,\approx \,34{pm}\,{V}^{-1},\)7\(.\) We adopt this theoretical value because experimental Maker-fringes measurements on bulk 3C-SiC is not available yet25,26,27. These elements mix three waves that are linearly polarized along three orthogonal crystal axes upon satisfying the energy conservation of \({\omega }_{p}={\omega }_{s}+{\omega }_{i}\), where \({\omega }_{p,s,i}\) are the angular frequencies of the pump, signal and idler. To minimize the number of waveguide eigenmodes involved in the nonlinear processes, we chose Type-I phase matching noting that 3C-SiC is non-birefringent. As shown in Fig. 1b, based on the (001) 3C-SiC film, we set the NIR input signal and the idler waves around 1550 nm to be transverse electric (TE)-polarized (parallel to the chip) with the x- and y-components of the fields, while the pump wave around 780 nm to be transverse magnetic (TM)-polarized with the z-component of the field. We must satisfy the phase-matching condition of \({\beta }_{p}={\beta }_{s}+{\beta }_{i}\), where \({\beta }_{p/s/i}\) are the propagation constants of the three waveguide modes.
Brendel, J., Gisin, N., Tittel, W. & Zbinden, H. Pulsed Energy-Time Entangled Twin-Photon Source for Quantum Communication. Phys. Rev. Lett. 82, 2594–2597 (1999).
She, X., Huang, A. Q., Lucia, O. & Ozpineci, B. Review of Silicon Carbide Power Devices and Their Applications. IEEE Trans. Ind. Electron. 64, 8193–8205 (2017).
The global cutting tool inserts market highlights some of the key market participants operating in the global cutting tool inserts market, such as Kennametal Inc., Sumitomo Electric Carbide Inc., Sandvik AB, Knight Carbide Inc., Compagnie de Saint-Gobain, Total Carbide Ltd., Asahi Diamond Industrial Co. Ltd., Tomei Diamond Co. Ltd., Kyocera Corp., Mitsubishi Materials Corp., Showa Denko K.K., YG-1 Co. Ltd., Element Six, Iscar Ltd. and NGK Spark Plugs Co. Ltd. (NTK Cutting Tools).
Xu, Y. et al. Mitigating photorefractive effect in thin-film lithium niobate microring resonators. Opt. Express 29, 5497 (2021).
Process of both external (e.g., thread milling) and internal (e.g., tapping, thread milling) cutting, turning and rolling of threads into particular material. Standardized specifications are available to determine the desired results of the threading process. Numerous thread-series designations are written for specific applications. Threading often is performed on a lathe. Specifications such as thread height are critical in determining the strength of the threads. The material used is taken into consideration in determining the expected results of any particular application for that threaded piece. In external threading, a calculated depth is required as well as a particular angle to the cut. To perform internal threading, the exact diameter to bore the hole is critical before threading. The threads are distinguished from one another by the amount of tolerance and/or allowance that is specified. See turning.
Lukin, D. M. et al. 4H-silicon-carbide-on-insulator for integrated quantum and nonlinear photonics. Nat. Photonics 14, 330–334 (2020).
Lu, X., Lee, J. Y., Rogers, S. & Lin, Q. Optical Kerr nonlinearity in a high-Q silicon carbide microresonator. Opt. Express 22, 30826 (2014).
a Illustration of the principle of generating time-bin entanglement using a free-space Franson unbalanced interferometer. Inset (i): Illustration of the three-time-bin histogram. b Measured histograms at two different piezo-voltage settings. c Measured single-channel counts with different piezo-voltage settings. d Measured coincidence counts of the central bin upon various piezo-voltage settings and fitted with a sinusoidal function.
Open Access This article is licensed under a Creative Commons Attribution 4.0 International License, which permits use, sharing, adaptation, distribution and reproduction in any medium or format, as long as you give appropriate credit to the original author(s) and the source, provide a link to the Creative Commons licence, and indicate if changes were made. The images or other third party material in this article are included in the article’s Creative Commons licence, unless indicated otherwise in a credit line to the material. If material is not included in the article’s Creative Commons licence and your intended use is not permitted by statutory regulation or exceeds the permitted use, you will need to obtain permission directly from the copyright holder. To view a copy of this licence, visit http://creativecommons.org/licenses/by/4.0/.
Geographically, APAC and European markets are picking up pace in the global cutting tool inserts market, owing to expansion of industrial infrastructure and an upsurge in the automotive and oil and gas industry over the years. Europe being an automotive hub has a lot of scope for transportation industry including railways. Most of the metal used in this industry is machined with cutting tool inserts, thus accounting for better sales of the same. The transportation segment is anticipated to soar the cutting tool inserts market in Europe. The demand for cutting tool inserts is majorly driven by its applications in various sectors such as aerospace, automotive, marine, medical, woodworking, die and mold, driven by growth in global GDP. Moreover, stable economic growth in developing countries such as India, Brazil, China and ASEAN countries, and rising urbanization and expenditure in these regions, acts as the major growth factors that are propelling the growth of the cutting tool inserts market.
Orais, N. M. et al. Directionally induced quasi-phase matching in homogeneous AlGaAs waveguides. Opt. Lett. 42, 4287–4290 (2017).
Lundquist, P. M., Lin, W. P., Wong, G. K., Razeghi, M. & Ketterson, J. B. Second harmonic generation in hexagonal silicon carbide. Appl. Phys. Lett. 66, 1883–1885 (1995).
Jiayang Li designed, simulated, fabricated and characterized the devices. Qianni Zhang and Jiantao Wang performed the characterization of the pump-to-SHG power dependence and the data analysis of SHG. Jiayang Li, Qianni Zhang, Jiantao Wang and Andrew W. Poon wrote the manuscript. Andrew W. Poon supervised this project.
Mariani, S. et al. Second-harmonic generation in AlGaAs microdisks in the telecom range. Opt. Lett. 39, 3062–3065 (2014).
Chang, L. et al. Strong frequency conversion in heterogeneously integrated GaAs resonators. APL Photonics 4, 036103 (2019).
Communications Physics thanks Florian Kaiser and the other anonymous, reviewer(s) for their contribution to the peer review of this work.
Li, J., Zhang, Q., Wang, J. et al. An integrated 3C-silicon carbide-on-insulator photonic platform for nonlinear and quantum light sources. Commun Phys 7, 125 (2024). https://doi.org/10.1038/s42005-024-01620-x
We fabricate the devices on the same integrated 3C-SiCoI platform as described in9. We use the commercially available polished 4” (001) 3C-SiC-on-Si wafers from NOVASIC. Before the wafer bonding, we deposit a layer of plasma-enhanced chemical vapor deposition (PECVD) SiO2 with a thickness of 500 nm on the 3C-SiC-on-Si dies (1 × 1 cm) to serve as a high-quality under-cladding layer. The PECVD recipe features SiH4 and N2O gas flows of 15 and 1410 sccm, respectively. The chamber is heated to 300 °C and kept at a pressure of 900 mTorr. The coil power is 30 W. We use the Karl Suss SB6 wafer bonder for the anodic bonding process between the dies and the soda lime glass substrate. We attain the bonding under 380 °C in a vacuum environment and upon an applied voltage of 1000 V. We use the standard 25% tetramethylazanium hydroxide solution to completely remove the silicon substrate at 80 °C and to expose the 3C-SiC film. The thickness of the 3C-SiC film is 1.5 μm initially. We thin down the film to our targeted thickness of ~800 nm by deep reactive ion etching (DRIE) process. The etching chemistry adopts SF6 and O2 gases with flows of 25 and 5 sccm, respectively. We kept the chamber pressure at 10 mTorr. The coil power and the platen power are 800 and 250 W, respectively. We then deposit a layer of the PECVD SiO2 as the hard mask. We pattern it with electron-beam lithography and with a standard SiO2 dry etching process available in the cleanroom. This SiO2 hardmask etching recipe adopts C4F8 and H2 gases with gas flows of 12 and 8 sccm, respectively. We kept the chamber pressure at 4 mTorr. The coil power and the platen power are 925 and 130 W, respectively. Then, we use the same SF6-based etching chemistry as in the film thinning-down process to dry etch the 3C-SiC patterns. To attain a steep sidewall slope, we add 3 s of C4H8 passivation steps after 5 s of dry etching steps to protect the waveguide sidewalls. The gas flow of C4H8 is 40 sccm and the coil power is 200 W. We recorded an etching selectivity of 3C-SiC:SiO2 ≈ 1.45. Afterwards, we deposit a layer of PECVD SiO2 with a thickness of 500 nm as the upper-cladding layer. Finally, we cleave the chip to expose the waveguide end-facets for coupling using long-working-distance (LWD) objective lenses.
Key applications of cutting tool inserts, such as threading, milling and shearing, parting and grooving, and drilling and boring, are expected to drive the cutting tool inserts market growth at a significant rate. The stainless steel segment is estimated to retain its market share over the forecast period. According to the report, the demand for cutting tool inserts is expected to be driven primarily by the rise of automotive industry, oil and gas sector, construction industry, urbanization, and the increasing demand from other end use industries. Furthermore, massive demand from automotive OEMs, automotive refinishing service companies, construction companies, general industrial manufacturers and maintenance service providers, marine service companies, manufacturers of cans, coils, and wood and transport industries will contribute to the demand for cutting tool equipment, which, in turn, will augment the demand for cutting tool inserts.
We estimate the on-chip power based on the power measured by an OSA considering the insertion losses of the lens-to-fiber coupling, the OSA and of the waveguide output end-facets. The measured insertion losses of the lens-to-fiber coupling and of the OSA are 3.0 dB and 2.4 dB, respectively. We then estimated the output power. The insertion loss of a test straight waveguide fabricated adjacent to the device is slightly below 12.0 dB for both the 780 nm and 1550 nm wavelengths. We neglect the waveguide propagation loss over this 1.2 mm long waveguide and adopted an average waveguide end-facet out-coupling loss as 5.8 dB as the maximum out-coupling loss, assuming the waveguide end-facet in-coupling loss is equal to or larger than the out-coupling loss, for estimating the on-chip power for both the 780 nm and 1550 nm light.
Bogdanov, S., Shalaginov, M. Y., Boltasseva, A. & Shalaev, V. M. Material platforms for integrated quantum photonics. Opt. Mater. Express 7, 111 (2017).
The global cutting tool inserts market is likely to account for ~US$ 18.1 Bn by the end of the assessment year 2019, and is estimated to expand at a CAGR of ~7.0% during the forecast period of 2019-2029. Among the type of material, the carbides segment is anticipated to grow at a noteworthy rate, owing to their cost effectiveness and durability, thereby contributing to the relatively high growth rate of the carbides segment over the forecast period.
Romijn, J. et al. Integrated Digital and Analog Circuit Blocks in a Scalable Silicon Carbide CMOS Technology. IEEE Trans. Electron Devices 69, 4–10 (2022).
Key strategies are the expansion of production capacities and focus on mergers and acquisitions to increase their global and regional footprint in the cutting tool inserts market. For instance, in July 2017, Sumitomo Electric Carbide Inc., started operations at the Dayton Plant, which is operated by one of its subsidiaries named Sumiden Wire Products Corp. (SWPC) in the U.S. The plant is dedicated to the manufacturing of wires for automobile springs. In April 2017, Kyocera Corp. expanded its industrial ceramic manufacturing business in Washington, U.S. ILJIN Diamond Co. Ltd. is focused on developing human friendly technologies that can impart to better customer relations and long-term deeds.
Lu, X., Lee, J. Y. & Lin, Q. High-frequency and high-quality silicon carbide optomechanical microresonators. Sci. Rep. 5, 1–9 (2015).

where \({N}_{{s}_{1}{s}_{2}i}\) is the triple-coincidence counts for both the beam-split signal channels and the heralding idler channel and \({N}_{{s}_{1(2)}i}\) are the coincidence counts between the one split signal channel and the heralding idler channel. Equation (4) indicates that the noise from the idler (heralding) channel has a dominant effect. To reduce the influence, we assign one channel of the low-noise SNSPD for heralding, while using another SNSPD channel and one extra avalanche photodetector for detecting signals 1 and 2, respectively.
This work is supported by the Research Grants Council of the Hong Kong Special Administrative Region, under Project 16202919. We thank Yuanbin Liu from Shanghai Jiao Tong University for helpful discussions.
When used in lathe or screw-machine operations, this process separates a completed part from chuck-held or collet-fed stock by means of a very narrow, flat-end cutting, or parting, tool.
In this work, we adopted the 3C-SiC as this is the only polytype that is epitaxially grown on wafer-scale silicon substrates. This is promising for future large-scale integration and wafer-scale fabrication. Moreover, an epitaxially grown 3C-SiC layer features a well-controlled thickness, which minimizes the non-uniformity in the SiC film thickness and avoids the material waste during the grinding process which is unavoidable in the case of utilizing a bulk 4H-SiC substrate20. We demonstrate on our 3C-SiCoI platform through anodically bonding the 3C-SiC film onto a glass substrate (see Methods). We design a waveguide-coupled elliptical microring resonator using type-I (signal and idler are linearly polarized orthogonally to the pump) MPM for \({\chi }^{(2)}\)-based nonlinear and quantum light sources. We design for the conversion between 780 nm near-visible wavelengths and the 1550 nm near-infrared (NIR) wavelengths. We demonstrate an SHG conversion efficiency of 17.4 ± 0.2% W−1 and difference-frequency generation (DFG) with a non-degenerate signal-idler bandwidth of up to 97 nm. We observe DFG across a bandwidth of 9.5 nm around pumping in the 780 nm wavelengths. We demonstrate a SPDC source with a photon-pair generation rate (PGR) of 4.8 MHz and a coincidence-to-accidental ratio (CAR) of \(3361\pm 84\) at an on-chip pump power of 5 mW. At this pump power, we obtain a low heralded single-photon second-order coherence \({g}_{H}^{\left(2\right)}=0.0007\) with a confidence interval of (0.0001, 0.002) with 68% confidence level using these photon-pairs. We generate a time-bin entanglement with a visibility of \(86.0\pm 2.4 \%\) in a free-space Franson interferometer23 using our photon-pair source, which was reported recently24. Our work shows the feasibility of SiC-based on-chip nonlinear and quantum light sources based on \({\chi }^{(2)}\) nonlinearity.
We insert the free-space interferometer into the down-stream of the SPDC experimental setup to prepare the entanglement. We pump at 787.7 nm with an estimated 5 mW on-chip power and measure the photon-pair channels of 1569/1581 nm. Figure 6b shows the measured histograms at two different piezo-voltage settings fine-tuning the interferometer long arm. The three-peak time-bins are separated by a time difference of 0.58 ns, corresponding to a path difference of 17.4 cm. The coincidence counts at the \({{|SS} > }_{{si}}\) and \({{|LS} > }_{{si}}\) time-bins are not sensitive to the piezo-voltage while the total coincidence counts in the central time-bin vary sinusoidally with the piezo-voltage. The quantum interference between the superposed photon-pair states \({{|SS} > }_{{si}}\) and \({{|LL} > }_{{si}}\) yields a sinusoidal modulation of the coincidence counts (assuming 50:50 beam splitters) as \(0.5{N}_{\max }[1+V\cos \left({\varphi }_{s}+{\varphi }_{i}\right)]\), where \({N}_{\max }\) and \(V\) are the maximum transmitted central time-bin counts and the visibility of the quantum interference. Figure 6c, d shows the measured channel counts of the signal/idler photons and the measured coincidence counts of the central time-bin, respectively. Each data point corresponds to a data collection spanning 30 s. We do not observe first-order (single-photon) quantum interference as the interferometer path difference is significantly longer than the photon cavity coherence time so the two wave-packets of the same photon simultaneously traveling in the two different interferometer arms cannot meet each other. We observe second-order quantum interference in the coincidence counts in the central time-bin. Using a sinusoidal fitting, we extract a visibility of \(V=86.0\pm 2.4 \%\). This is significantly exceeding the Bell’s inequality limit of \(70.1 \%\)23\(,\) indicating a clear time-bin entanglement. We attribute the non-ideal visibility to the drifts of the optical alignment and collimation conditions of the two interferometer arms during the experiment. We note that our Franson interferometer is not actively stabilized, and thus phase drift is potentially the main source of fringe visibility degradation. In our future work, we could employ active stabilization on the interferometer to overcome the issue. We note that the superposed \({{|SS} > }_{{si}}\) and \({{|LL} > }_{{si}}\) states do not have the same probability amplitudes in practice due to different setup insertion losses for the two interferometer arms.
Nonetheless, none of the crystalline SiC polytypes offers a large refractive index contrast with their native substrates. Photonic devices on SiC are thus often fabricated on suspended SiC films12,13,14,15 and on the SiCoI platforms11,16,17,18,19,20,21. Integrated SiCoI platforms rely on molecular bonding16,17,18,19,20,21 or anodic bonding11 to transfer the SiC films onto SiO2 substrates, which enables a better scalability for larger-scale integrated photonic circuits. The optical nonlinearity of SiC have been studied last decade13,15. Optical parametric oscillation and soliton generation are demonstrated based on high-quality-factor (high-Q) (\( > {10}^{6}\)) microresonators on a 4H-SiCoI platform17,18 while four-wave mixing is demonstrated on a suspended 3C-SiC platform with relatively lower Q factors (\(\sim 7400\))14. The demonstration of \({\chi }^{(2)}\) nonlinearity-based sources remains a challenge partly due to the difficulty to attain the phase-matching condition across an octave while engineering the waveguide dispersion. Recently, second-harmonic generation (SHG) with efficiencies of 360% W−1 and 3.91% W−1 were demonstrated in 4H-SiCoI using modal phase matching (MPM) and a suspended 4H-SiC microdisk19,20, respectively. A time-averaged efficiency of 15% W−1 using a pulsed laser was demonstrated by using a suspended 6H-SiC photonic-crystal cavity13. Moreover, Pockels-effect-based microring modulators have been demonstrated on both 4H- and 3C-SiCoI platforms21,22. To our knowledge, spontaneous parametric down conversion (SPDC)-based photon-pair sources have not been demonstrated on SiC platforms.
We accumulate more than 6 h of data (40 Gb) and obtain only 1 triple coincidence count \({N}_{{s}_{1}{s}_{2}i}\) while collecting \({N}_{{s}_{1}i}={{{{\mathrm{1,949,638}}}}}\), \({N}_{{s}_{2}i}={{{{\mathrm{441,670}}}}}\) and \({N}_{i}={{{{\mathrm{610,078,920}}}}}\). Assuming a Poissonian distribution40 of the triple coincidence event, we estimate a low \({g}_{H}^{(2)}\) = 0.0007 and a confidence interval of (0.0001, 0.002) within approximately one standard deviation. This is one order of magnitude below recently reported work using second- and third-order SPDC processes38,39,40,41. Hence, our device can function as low-noise heralded single-photon sources.
Yamada, S. et al. Second-harmonic generation in a silicon-carbide-based photonic crystal nanocavity. Opt. Lett. 39, 1768 (2014).
A few conventional phase-matching techniques, including periodic-poling-based quasi-phase-matching (QPM)28,29, birefringence-based phase-matching30 and cyclic QPM31, are not available for the 3C-SiC material. We chose to design for a MPM, where we adopted a higher-order TM20 mode at the pump wavelength to match the effective refractive index of the fundamental TE00 mode at the signal/idler NIR wavelengths.
We fabricate the device in a cleanroom (see Methods). We characterize the waveguide transmission and SHG from a waveguide-coupled elliptical microring (see experimental details in Supplementary Note 4). The operating device has a designed waveguide of 1050 nm. Given the uncertainty of the actual \({n}_{{{{{{\rm{eff}}}}}}}\) induced by the continuously varying waveguide curvature within the elliptical cavity and the variance of the waveguide width caused by the fabrication process, we consider this device width a close match to the simulated waveguide width for MPM. Figure 3a shows the scanning electron microscopy (SEM) image of the device. Figure 3b, c shows the SEM images in zoom-in view of the sidewall and in the cross-sectional view of the waveguide end-facet, respectively. We extract a root-mean-square (RMS) of the waveguide sidewall roughness of ~7.3 nm based on analysis of the top-view SEM image (see Supplementary Note 5). This RMS is significantly larger than the previously reported value on 3C-SiC16. Figure 3d shows the near-field image measured at the through waveguide end facet by a charge-coupled device (CCD) camera upon a cavity resonance at 787.7 nm. It shows three intensity maxima, suggesting the cavity resonance has an TM20 waveguide mode while the higher-order mode in the through waveguide could be redistributed due to the waveguide tapering. The actual distribution Fig. 3e shows the corresponding lens-to-lens normalized transmission spectra of the device under a stabilized stage temperature of \(22^\circ C\). The measured transmission spectra in the 780 nm and 1550 nm wavelengths show a good double-resonance alignment for SHG between the resonances of 787.7 nm and 1575.4 nm, as indicated by the zoom-in view of Fig. 3f. The loaded Q factors of the 1575.4 nm and of the 787.7 nm resonances are around \(2.5\times {10}^{4}\) and \(1.4\times {10}^{4}\), respectively. This is lower than the \({Q}_{{loaded}} > {10}^{5}\) we previously reported11. By a Lorentzian resonance lineshape fitting, shown as the magenta dashed line in Fig. 3f, we obtain an intrinsic Q factor of ~3.0 × 104 and thereby extract a waveguide loss of ~11 dB cm−1 at 1574 nm. The free spectral range (FSR) of the 1575.4 nm and the 787.7 nm resonances are ~6.9 nm (~800 GHz spacing) and ~1.5 nm, respectively. We conduct the waveguide dispersion analysis of the resonance (see Supplementary Note 6).
Guidry, M. A., Lukin, D. M., Yang, K. Y., Trivedi, R. & Vučković, J. Quantum optics of soliton microcombs. Nat. Photonics 16, 52–58 (2022).
Wang, R., Li, J., Cai, L. & Li, Q. “Investigation of the electro-optic effect in high-Q 4H-SiC microresonators,”. Opt. Lett. 48, 1482–1485 (2023).
a Measured single-photon flux detected by the SNSPDs with different DWDM channels. SNSPDs: superconducting nanowire single-photon detectors. DWDM channels: dense wavelength-division multiplexed channels. FSR: free spectral range. b, c Measured histograms of the relative arrival times of the first adjacent resonances at 1569/1581 nm and of the second adjacent resonances at 1563/1588 nm, respectively. The histograms are measured multiple times to obtain the error bars for all the bins. Measured power dependence of: (d) the single-channel counts, (e) the photon-pair coincidence counts, (f) the estimated PGRs, and of (g) the estimated CARs. The error bars are the standard deviations of (b–e) the coincidence counts, (f) PGRs and of (g) CARs.
where \({\epsilon }_{0}\) is the vacuum permittivity and \({\epsilon }_{1(2)}=6.55(6.81)\) is the relative permittivity of 3C-SiC at 1550 nm and 780 nm11, respectively (See Supplementary Note 8 for details). We estimate a nonlinear coupling of \(\gamma \,\approx \,7.6\times {10}^{5}{Hz}\). From (1), assuming a double-resonance condition, we assume \({\varDelta \omega }_{1(2)} \,\ll \,{\kappa }_{1,t}\). Given our measured loaded-Q factors at 1550 nm and 780 nm and assuming an external coupling \({\kappa }_{1(2),e} \,\approx \,{\kappa }_{1(2),t}/2\) we theoretically estimated \({\eta }_{{SHG}} \,\approx \,19 \% {W}^{-1}\), which is consistent with our experimental estimation.
We describe the experimental setup in Supplementary Note 4. We fix the pump wavelength \({\lambda }_{p}\) at a cavity resonance at 787.7 nm and measure the SPDC with two-channel superconducting nanowire single-photon detectors (SNSPDs). The SNSPDs feature dark counts of ~100 Hz for each of the two channels. Figure 5a shows the measured single-photon flux detected by the SNSPDs with different dense wavelength-division multiplexed (DWDM) channels that span the corresponding cavity resonance wavelengths in the NIR. The estimated on-chip pump power is 5.8 mW, which is the maximum on-chip power obtainable from our setup using a continuous-wave semiconductor laser. We register the photon arrival times from two non-degenerate photon-pairs, namely 1569/1581 nm and 1563/1588 nm, by the time-digit converter (TDC) with a resolution of 5 ps per bin. Figure 5b, c shows the measured histograms of the relative arrival times of the two pairs of channels at a pump power of 5 mW, which feature strong coincidence. We record 60 s of data for each pair for statistics. We adopted a double-side exponential decay relation \({N}_{{si}}(t)={N}_{0}{e}^{-\left|t\right|/\tau }\) to fit the data, where \({N}_{{si}}(t)\), \({N}_{0}\) and \(\tau\) are the coincidence count in the time-bin at delay \(t\), the maximum coincidence count at zero-time delay and the photon life-time. The fitted value of \(\tau =26\pm 1{{{{{\rm{ps}}}}}}\) for both the pairs correspond to the loaded Q factor of ~\(2.5\times {10}^{4}\) in the NIR wavelengths, which is consistent with our transmission resonance measurements (see Fig. 3e). We define the coincidence counts summed up from the 12 bins within the \(\pm \tau\) range of the histograms as the total coincidence count \({N}_{{si}}\).
Guo, X. et al. Parametric down-conversion photon-pair source on a nanophotonic chip. Light Sci. Appl. 6, 1–8 (2017).
Yamaoka, S. et al. Directly modulated membrane lasers with 108 GHz bandwidth on a high-thermal-conductivity silicon carbide substrate. Nat. Photonics 15, 28–35 (2021).
Table 1 summarizes the state-of-the-art resonance-enhanced SHG results from different SiC platforms13,19,20. Our SHG conversion efficiency on 3C-SiCoI is lower than the best value in 4H-SiCoI because of the limited Q factors of our platform at this stage. We characterize the same device for DFG and SPDC processes.
Dorin, B. & Ye, W. Two-mode division multiplexing in a silicon-on-insulator ring resonator. Opt. Express 22, 4547–4558 (2014).
European investments in Asia are motivated by the need to reduce costs, to exploit benefits of the local supply chains and to be close to an untapped customer base, which enables them to better understand user needs and better serve their customers. European machine tool builders’ investment strategies abroad vary from strategic alliances to joint ventures, from the acquisition of foreign companies to opening production facilities in third countries. Increasing investments of global car manufacturers in emerging countries, for instance, India, China and Brazil, along with large publicly funded energy and infrastructure projects in these countries, make them attractive enough for European investments from machine tool builders.
Wang, C. et al. High-Q microresonators on 4H-silicon-carbide-on-insulator platform for nonlinear photonics. Light Sci. Appl. 10, 139 (2021).
Li, J. & Poon, A. W. A 3C-SiC-on-Insulator-Based Integrated Photonic Platform Using an Anodic Bonding Process with Glass Substrates. Micromachines 14, 399 (2023).
Powell, K. et al. High-Q suspended optical resonators in 3C silicon carbide obtained by thermal annealing. Opt. Express 28, 4938 (2020).
Kwon, O., Park, K.-K., Ra, Y.-S., Kim, Y.-S. & Kim, Y.-H. Time-bin entangled photon pairs from spontaneous parametric down-conversion pumped by a cw multi-mode diode laser. Opt. Express 21, 25492 (2013).
We first fix the pump wavelength at 780 nm and assume degenerate signal and idler wavelengths at 1560 nm. Figure 2a shows the numerically simulated electric-field amplitude distributions of a TE00 mode at 1560 nm and a TM20 mode at 780 nm, respectively, using a finite-difference eigenmode tool. To search for the potential waveguide geometry to match the effective refractive index of the two modes, we systematically vary the thickness and the width of the rib waveguide structure. Based on our previous device fabrication results, we assumed a symmetric waveguide sidewall slope of \(86^{\circ}\). We kept a thin 3C-SiC slab of 100 nm un-etched to avoid undercutting the SiO2 layer underneath the SiC waveguide. The 3C-SiC is clad by a SiO2 layer with a thickness of 500 nm. We adopted a Cauchy model with A, B coefficients of 2.553 and 0.03539 to calculate the material index of 3C-SiC. To simplify the simulation and design, we assume a straight waveguide structure. Figure 2b shows the numerically simulated effective refractive indices of the two modes with different 3C-SiC film thicknesses as a function of the waveguide width. The width corresponds to the top side of the trapezoidal waveguide cross-section. We can attain the MPM with a waveguide width of 1000 nm and a thickness of 800 nm. The MPM width increases with the 3C-SiC film thickness while the mismatch between the effective refractive indices becomes more tolerant to the waveguide width variation with a thick film.
Severino, A. 3C-SiC epitaxial growth on large area silicon: Thin films. Silicon Carbide Epitaxy 37, 145–191 (2012).
Sato, H., Shoji, I., Suda, J. & Kondo, T. Accurate measurements of second-order nonlinear-optical coefficients of silicon carbide. Mater. Sci. Forum 615 617, 315–318 (2009).
We designed the waveguide dimensions at a thickness of 800 nm and a top width of 1000 nm, with a slab thickness of 100 nm. Figure 2c shows the numerically simulated non-degenerate DFG effective refractive indices \({n}_{{eff}}\) of the modes with wavelengths. Figure 2d shows the numerically simulated non-degenerate DFG phase-matching condition map with the non-degeneracy \(\left({f}_{s}-\frac{{f}_{p}}{2}\right)\) between the signal/idler frequencies (satisfying energy conservation) over the pump wavelength, following Fig. 2c. Here, \({f}_{s(p)}\) is the frequency of the signal (pump) light, respectively. We adopted a total accumulated phase-mismatch of \(\left|\Delta \beta L\right|=\left|({\beta }_{p}-{\beta }_{s}-{\beta }_{i})L\right| < \pi\) within the cavity as a working criterion for defining the MPM region. We notice a pumping wavelength range >10 nm and a non-degeneracy >25 THz. However, a \(\pm 50{nm}\) of the waveguide width will significantly red- or blue-shift the MPM wavelength window beyond the \(1.5 \sim 1.6\mu m\) wavelengths (see Supplementary Note 2). Finally, we adopted a bus waveguide with the same width of 1000 nm coupled to the microring cavity to input-couple the TE00 mode at NIR wavelengths while utilizing a drop waveguide with a narrower width of 400 nm to phase-match the TM00 mode in the bus waveguide and in the TM20 mode in the microring cavity. The coupling gap spacing is 200 nm for both the coupled waveguides. The interaction length is \(15\mu m\) for the through waveguide and is \(30\mu m\) for the drop waveguide. Both the waveguides are widened to a width of \(4\mu m\) for butt-coupling. Supplementary Note 3 details the device layout and the numerical simulations of the device.
Guidry, M. A. et al. Optical parametric oscillation in silicon carbide nanophotonics. Optica 7, 1139 (2020).
Table 2 summarizes the state-of-the-art microring-based quantum light sources from different second-order nonlinear platforms38,39. Although our PGR is limited by our Q factors, we have demonstrated a low-noise performance with the lowest \({g}_{H}^{\left(2\right)}\) among all the platforms.
Publisher’s note Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.
Assuming \({\kappa }_{p,e}\,\approx \,{\kappa }_{p,t}/2\), we obtain from Eq. (3) the theoretical PGR efficiency of \(\frac{{PGR}}{{P}_{p}}=2.3{{{{{\rm{MHz}}}}}}{{{{{{\rm{mW}}}}}}}^{-1}\), which is close to our measured result. Our estimated PGR values are smaller than those reported values of the AlN platform38 and of the LNoI platform39. We attribute this to our un-optimized device fabrication process, as the pair-generation efficiency is primarily determined by the total cavity loss (see Eq. (3)). Figure 5g shows the CAR of the two pairs, given by \({CAR}=\frac{{N}_{{si}}}{{N}_{{{{{{\rm{noise}}}}}}}}\). Here we integrate the rare coincidence counts 1 ns away from the central peak of the coincidence counts to obtain an average coincidence count per bin and multiply the 12 number of bins adopted in the summation of \({N}_{{si}}\) to obtain the \({N}_{{{{{{\rm{noise}}}}}}}\) (see Supplementary Note 11). We obtain a high \({CAR}=3361\pm 84\) upon pumping at the on-chip power of 5 mW from the 1569/1581 nm photon-pairs. We also characterize the SPDC process using another device with a designed waveguide width of 1000 nm (see Supplementary Note 12). As indicated by the DFG process, this device generates photon-pairs in the C-band channels.
Silicon carbide (SiC) polytypes are emerging for integrated nonlinear and quantum photonics due to their wide-bandgap energies, second-order optic nonlinearity and process compatibility with complementary metal-oxide-semiconductor technologies. Among polytypes, 3C-SiC is the only one epitaxially grown on wafer-scale silicon substrates. However, on-chip nonlinear and quantum light sources leveraging the second-order nonlinearity of 3C-SiC have not been reported to our knowledge. Here, we design and fabricate an elliptical microring on 3C-SiC. We demonstrate a nonlinear light source with a second-harmonic generation efficiency of \(17.4\pm 0.2 \% {W}^{-1}\) and difference-frequency generation with a signal-idler bandwidth of 97 nm. We demonstrate a spontaneous parametric down-conversion source with a photon-pair generation rate of 4.8 MHz and a coincidence-to-accidental ratio of \(3361\pm 84\). We measure a low heralded single-photon second-order coherence \({g}_{H}^{\left(2\right)}=0.0007\). We observe time-bin entanglement with a visibility of \(86.0\pm 2.4 \%\) using this source. Our work paves a way toward SiC-based on-chip nonlinear and quantum photonic circuits.
Zielinski, M. et al. Structural Quality, Polishing and Thermal Stability of 3C-SiC/Si Templates. Mater. Sci. Forum 924, 306–309 (2018).
Wang, L. et al. Growth of 3C-SiC on 150-mm Si (100) substrates by alternating supply epitaxy at 1000 °C. Thin Solid Films 519, 6443–6446 (2011).
Ma, C. et al. Silicon photonic entangled photon-pair and heralded single photon generation with CAR > 12,000 and g(2)(0) < 0.006. Opt. Express 25, 32995 (2017).
The silicon carbide (SiC) semiconductor industry is expanding rapidly driven by the growing needs for high-power electronics1. Meanwhile, integrated photonic platforms based on SiC polytypes are emerging in recent years, including hexagonal (4H-, 6H-) SiC and cubic (3C-) SiC. They offer a wide bandgap energy from 2.3 to 3.2 eV2, which enables transmissions of visible light and does not suffer from nonlinear two-photon absorption at the 1550/1310 nm telecommunications wavelengths. They also feature a high hardness for photon-phonon interactions3, a fast heat dissipation for high-speed directly modulated lasers4 and different color centers for single quantum emitters5,6. Owing to their non-centrosymmetric crystal lattices, SiC polytypes feature second-order nonlinear susceptibilities \({\chi }^{(2)}\). For example, 3C-SiC features \({\chi }_{{zxy}}^{(2)}\approx\) 34 pm V−1 (theoretical)7 while 4H-SiC features \({\chi }_{{zzz}}^{(2)}\approx\) −23.4 pm V−1 and \({\chi }_{{zxx}}^{(2)}\approx\) 13.0 pm V−1 8 This enables nonlinear and spontaneous quantum light sources based on \({\chi }^{(2)}\) optic processes on integrated SiC-on-insulator (SiCoI) platforms. Compared with the integrated lithium niobate-on-insulator (LNoI) platform, SiC offers process compatibility with the mature wafer-level complementary metal-oxide-semiconductor compatibility (CMOS) processes and an insignificant photorefractive effect9,10,11. Supplementary Table 1 (see Supplementary Note 1) summarizes and compares conventional integrated second-order nonlinear photonic platforms.
For instance, wood working industry in Latin America is at a boom and this has facilitated the increase in the number of sales of cutting tool inserts. Cutting tool inserts are used to draw grooves in wooden furniture and artifacts. The most common type of cutting tool insert used to machine wood is carbide. In addition, with rising competition in the field of aerospace and marine, the requirement of jet engines, turbines, transmission parts and other vital components of aircraft or submarine has significantly increased the need for machining these components thereby increasing the scope of cutting tool inserts market.
Thank you for visiting nature.com. You are using a browser version with limited support for CSS. To obtain the best experience, we recommend you use a more up to date browser (or turn off compatibility mode in Internet Explorer). In the meantime, to ensure continued support, we are displaying the site without styles and JavaScript.
Xue, G. T. et al. Ultrabright Multiplexed Energy-Time-Entangled Photon Generation from Lithium Niobate on Insulator Chip. Phys. Rev. Appl. 15, 1 (2021).
Martini, F. & Politi, A. Four wave mixing in 3C SiC ring resonators. Appl. Phys. Lett. 112, 1–5 (2018).
Machining operation in which metal or other material is removed by applying power to a rotating cutter. In vertical milling, the cutting tool is mounted vertically on the spindle. In horizontal milling, the cutting tool is mounted horizontally, either directly on the spindle or on an arbor. Horizontal milling is further broken down into conventional milling, where the cutter rotates opposite the direction of feed, or “up” into the workpiece; and climb milling, where the cutter rotates in the direction of feed, or “down” into the workpiece. Milling operations include plane or surface milling, endmilling, facemilling, angle milling, form milling and profiling.
a–f Measured difference-frequency generation (DFG) spectra with different input pump and signal resonances. Different colors represent various groups of pump, signal and generated idler wavelengths. g Scatter plot of the triple-resonance-enhanced DFG modes. The region between the two dashed lines corresponds to the simulated MPM zone in Fig. 2d. h Measured power-dependence of the DFG with varying pump and observed signal powers.
Wang, J. et al. Bright room temperature single photon source at telecom range in cubic silicon carbide. Nat. Commun. 9, 4106 (2018).
Jiang, X., Wu, H. & Dai, D. Low-loss and low-crosstalk multimode waveguide bend on silicon. Opt. Express 26, 17680 (2018).




 18581906093
18581906093